Remarks on the Upper Centralc Series of a Group | Glasgow Mathematical Journal | Cambridge Core
- ️Wed Feb 19 2025
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Following, for example, Kurošs [8], we define the (transfinite) upper central series of a group G to be the series
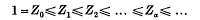
such that Zα + 1/Za is the centre of G/Zα, and if β is a limit ordinal, then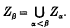 
If α is the least ordinal for which Zα =Zα+1=…, then we say that the upper central series has length α, and that Zα= His the hypercentre of G. As usual, we call G nilpotent if Zn= Gfor some finite n.
Type
Copyright © Glasgow Mathematical Journal Trust 1956
References
4.Duguid, A. M. and McLain, D. H., FC-soluble and FC-nilpotent groups, Proc. Comb. Phil.Soc., 52 (1956), 391–398.CrossRefGoogle Scholar
6.Hall, P., A contribution to the theory of groups of prime power order, Proc. Lond. Math.Soc., 36 (1933), 29–95.Google Scholar
7.Hall, P., Finiteness conditions for soluble groups, Proc. Lond. Math. Soc., (3) 4 (1954), 419–436.Google Scholar
9.Mal'cev, A. I., Generalised nilpotent algebras and their associated groups, Mat. Sbornik N.S., 25 (1949), 347–366. (in Russian).Google Scholar
11.Weir, A. J., The Sylow p-subgroups of the general linear group over finite fields of characteristic p, Proc. Amer. Math. Soc., 6 (1955), 454–464.Google Scholar